Math practice for 13 year olds - page 353 of 362
Number of problems found: 7234
- Radius
Determine the circle's radius if its perimeter and area are the same numbers. - Trapezoid
Are diagonals in a rectangular trapezoid perpendicular and bisect the angles? - Proof PT
Can you easily prove Pythagoras' theorem using Euclidean theorems? If so, do it. - Newton's task
Grass grows in the meadow equally fast and evenly. It is known that 99 cows graze meadow for 14 days and 95 cows by 22 days. How many cows graze the meadow for 77 days?
- Divisors
Find all divisors of number 432. How many are there? - Motion2
The cyclist started off town at 25 km/h. The car started after 1.9 hours behind him in the same direction. It caught up with him for 55 minutes. How fast and how long did the car run from the city to catch cyclists? - Wire D
Wire length 1 m is bent to form a semicircle circuit (including the diameter). Determine the radius of the semicircle. - Steps
How many steps do you save if you go square estate for diagonal (crosswise) rather than circumvent the two sides of its perimeter with 458 steps? - Estate
Semicircle estate must be a fence. The straight section has a 32 meters long fence. How many meters of the fence should we buy?
- Square 2
Points D[10,-8] and B[4,5] are opposed vertices of the square ABCD. Calculate the area of the square ABCD. - Square
Points A[9,9] and B[-4,1] are adjacent vertices of the square ABCD. Calculate the area of the square ABCD. - Gimli Glider
Aircraft Boeing 767 lose both engines at 35000 feet. The plane captain maintains optimum gliding conditions. Every minute, lose 2100 feet and maintain constant speed 201 knots. Calculate how long it takes for a plane to hit the ground from engine failure. - Triangle TBC
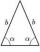TBC is an isosceles triangle with base TB with base angle 63° and legs length |TC| = |BC| = 25. How long is the base TB? - Cubes
One cube is an inscribed sphere, and the other one is described. Calculate the difference of volumes of cubes if the difference of surfaces in 231 cm².
- Cone A2V
The cone's surface in the plane is a circular arc with a central angle of 126° and an area of 415 cm². Calculate the volume of a cone. - Axial section
The axial section of the cone is an equilateral triangle with an area 208 m². Calculate the volume of the cone. - Rotation
The right triangle with legs 11 cm and 18 cm rotates around the longer leg. Calculate the volume and surface area of the formed cone. - Discount
The product has been discounted twice by 14%. What is the total discount given? - Hole
We will drill the cylinder shape hole in the cube's center with an edge 14 cm. The volume of the hole must be 27% of the cube. What should drill diameter be chosen?
1/3 of the area of the roof-shaped regular tetrahedral pyramid with base edge 10 m and height of 4 m is already covered with roofing. How many square meters still need to be covered?Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
