Circumference 7143

Peter drew a regular hexagon, the vertices of which lay on a circle 16 cm long. Then, for each vertex of this hexagon, he drew a circle centered on that vertex that ran through its two adjacent vertices.

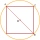
The unit was created as in the picture. Find the circumference of the marked flower.

Correct answer:

o =  32 cm

Step-by-step explanation:

$$\begin{gathered} x=16\text{cm} \\ r=x/(2\pi )=16/(2\cdot 3.1416)\doteq 2.5465\text{cm} \\ A=60{}^{\circ } \\ B=2\cdot A=2\cdot 60=120{}^{\circ } \\ {o}_1=2\pi \cdot r=2\cdot 3.1416\cdot 2.5465=16\text{cm} \\ {o}_2=\frac{B}{360}\cdot o_1=\frac{120}{360}\cdot 16=\frac{120\cdot 16}{360}=\frac{1920}{360}=\frac{16}{3}\doteq 5.3333\text{cm} \\ {o}_3=o_2/2=\frac{16}{3}/2=\frac{16}{3\cdot 2}=\frac{16}{6}=\frac{8}{3}\doteq 2.6667\text{cm} \\ o=12\cdot o_3=12\cdot \frac{8}{3}=\frac{12\cdot 8}{3}=\frac{96}{3}=32=32\text{cm} \\ o=4\pi \cdot r \end{gathered}$$

Try another example