Tangent - practice problems - page 8 of 15
Number of problems found: 292
- Power line pole
From point A, the power pole is visible at an angle of 18 degrees. From place B, which we reach if we go from place A 30m towards the pillar at an angle of 10 degrees. Find the height of the power pole. - Raindrops
The train runs at a speed of 14 m/s, and raindrops draw lines on the windows, forming an angle of 60 degrees with the horizontal. What speed do drops fall? - Quadrilateral prism
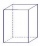Calculate the volume (V) and the surface (S) of a regular quadrilateral prism whose height is 28.6 cm and the deviation of the body diagonal from the base plane is 50°. - Steps
Find the height between the two floors if you know that the number of steps between the two floors is 18, the gradient is 30º, and the step length is 28.6 cm. Report the result in centimeters to the nearest centimeter.
- Angle of cone
The cone has a base diameter of 1.5 m. The angle at the central apex of the axial section is 86°. Calculate the volume of the cone. - A drone
A flying drone aimed the area for an architect. He took off perpendicularly from point C to point D. He was 300 m above ABC's plane. The drone from point D pointed at a BDC angle of 43°. Calculate the distance between points C and B in meters. - Cone side
Calculate the volume and area of the cone whose height is 10 cm, and the axial section of the cone has an angle of 30 degrees between height and the cone side. - Octagonal pyramid
Find the volume of a regular octagonal pyramid with height v = 100 and the angle of the side edge with the base plane is α = 60°. - Tetrahedral pyramid
Determine the surface of a regular tetrahedral pyramid when its volume is V = 120 and the angle of the sidewall with the base plane is α = 42° 30'.
- Coordinates of square vertices
I have coordinates of square vertices A / -3; 1/and B/1; 4 /. Find coordinates of vertices C and D, C and D. Thanks, Peter. - Isosceles triangle 10
In an isosceles triangle, the equal sides are 2/3 of the length of the base. Determine the measure of the base angles. - Hole's angles
I am trying to find an angle. The top of the hole is .625", and the bottom of the hole is .532". The hole depth is .250". What is the angle of the hole (and what is the formula)? - 3 phase load
Two wattmeters are connected to measure power in a 3 phase balanced load. Determine the total power and power factor if the two wattmeters read 1000 watts each (1) both positive and (2) the second reading is negative. - Angles of elevation
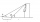From points A and B on level ground, the angles of elevation of the top of a building are 25° and 37°, respectively. If |AB| = 57m, calculate, to the nearest meter, the distances of the top of the building from A and B if they are both on the same side of
- The Eiffel Tower
The top of the Eiffel Tower is seen from 600 meters at a 30 degree angle. Find the tower's height. - Depth angles
At the top of the mountain stands a castle with a tower 30 meters high. We see the crossroad at a depth angle of 32°50' and the heel at 30°10' from the top of the tower. How high is the top of the mountain above the crossroad? - Calculate 9701
In the triangle, the side length AB = 6 cm, the height per side c = 5 cm, and the angle BCA = 35°. Calculate sides a b. - Northeasterly 9681
A military unit marches in a northerly direction from point A to point B, 15 km away. From place B, it goes 12 km in a northeasterly direction to place C. Determine the direct distance of cities A and C and certainly the deviation -alpha- by which the uni - One side
One side is 36 long with a 15° incline. What is the height at the end of that side?
The given is a regular hexagonal prism ABCDEFGHIJKL, which has all edges of the same length. Find the degree of the angle formed by the lines BK and CL in degrees.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
