Line segment - practice problems - page 5 of 9
Number of problems found: 174
- Length 20353
The given line is MN with a length of 11 cm. Change its length in the ratio: a) k = 2:1 b) k = 1:2 c) k = 17:11 d) k = 22:33 - Divides 20343 The KL line is 12 cm long. Point X divides the line in a ratio of 1:5. What is the length of line XL if point X is closer to point K?
- Outside tangents
Calculate the length of the line segment S1S2 if the circles k1 (S1, 8cm) and k2 (S2,4cm) touch the outside. - Chord BC
A circle k has the center at the point S = [0; 0]. Point A = [40; 30] lies on the circle k. How long is the chord BC if the center P of this chord has the coordinates [- 14; 0]?
- Coordinates of a centroind
Let A = [3, 2, 0], B = [1, -2, 4], and C = [1, 1, 1] be 3 points in space. Calculate the coordinates of the centroid of △ABC (the intersection of the medians). - Suppose
Suppose you know that the length of a line segment is 15, x2=6, y2=14, and x1= -3. Find the possible value of y1. Is there more than one possible answer? Why or why not? - A cell tower
A cell tower is located at coordinates (-5, -7) and has a circular range of 12 units. If Mr. XYZ is located at coordinates (4,5), will he be able to get a signal? - Rectangular 13731
I have a rectangular trapezoid ZIMA (the right angle at the top of Z. ZIMA = winter in English) ZI-7cm, ZM-5cm, AM-3.5cm, and I have to write the procedure and perform a test in the design task - Distance between 2 points
Find the distance between the points (7, -9), (-1, -9)
- Hexagon
Divide a regular hexagon into lines with nine completely identical parts; none of them must be in a mirror image (you can only rotate individual parts arbitrarily). - Set of coordinates Consider the following ordered pairs that represent a relation. {(–4, –7), (0, 6), (5, –3), (5, 2)} What can be concluded about the domain and range for this relation? A. The domain is the y values of the ordered pairs. B. The range is the set of output v
- ABCDEFGHIJKL 8426
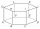The given is a regular hexagonal prism ABCDEFGHIJKL, which has all edges of the same length. Find the degree of the angle formed by the lines BK and CL in degrees. - Divide 8257
Divide a line 9 cm long in a ratio of 3:5:4 - Midpoint of the line segment
Length of lines MG = 7x-15 and FG = 33 Point M is the midpoint of FG. Find the unknown x.
- Midpoint 6 For line segment length is given: FM=8a+1, FG=42. Point M is the midpoint of FG. Find unknown a.
- Midpoint 5 FM=3x-4, MG=5x-26, FG=? Point M is the midpoint of FG. Use the given information to find the unknown measure or value.
- Find midpoint
FM=5y+13, MG=5-3y, FG=? M is the midpoint of FG. Use the given information to find the missing measure or value. - Diagonals at right angle
In the trapezoid ABCD, this is given: AB=12cm CD=4cm And diagonals crossed under a right angle. What is the area of this trapezoid ABCD? - Vertices of a right triangle
Show that the points D(2,1), E(4,0), and F(5,7) are vertices of a right triangle.
On side AB of triangle ABC, points D and E are given such that |AD| = |DE| = |EB|. Points A and B are the midpoints of segments CF and CG. Line CD intersects line FB at point I, and line CE intersects line AG at point J. Prove that the intersection of linDo you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
