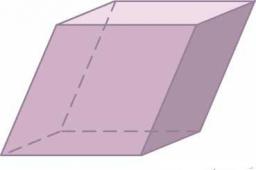
Prism
Calculate the volume of the rhombic prism. The prism base is a rhombus whose one diagonal is 34 cm, and the edge of the base is 20 cm. The edge length and height of the base of the prism are 4:5.
Correct answer:
Tips for related online calculators
Do you want to convert length units?
Tip: Our volume units converter will help you convert volume units.
See also our trigonometric triangle calculator.
Tip: Our volume units converter will help you convert volume units.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
Units of physical quantities:
Grade of the word problem:
Related math problems and questions:
- Rhombus base
Calculate the volume and surface area of prisms whose base is a rhombus with diagonals u1 = 12 cm and u2 = 15 cm. The prism height is twice the base edge length. - Calculate 2558
Calculate the size of the solid diagonals of a prism with a rhombus base if the sizes of the base diagonals are 16 cm and 20 cm and the height of the prism is 32 cm. Calculate the size of the base edge. - Quadrilateral 23881
Calculate the height of a regular quadrilateral prism whose base is a rhombus. The edge in the base is 7 cm long, the opposite edges are 5 cm apart, and we also know that the entire body has a volume of 1dm³. - Calculate 23411
The prism with a diamond base has one base diagonal of 20 cm and a base edge of 26 cm. The edge of the base is 2:3 to the height of the prism. Calculate the volume of the prism.
- Support colum
Calculate the support column's volume and surface. It is shaped as a vertical quadrangular prism whose base is a rhombus with diagonals u1 = 102 cm and u2 = 64 cm. The column height is 1. 5m. - Box
The cardboard is a box-shaped quadrangular prism with a rhombic base. Rhombus has a side 5 cm, one diagonal 8 cm long, and the box's height is 12 cm. The package will open at the top. How many cm² of cardboard do we need to cover overlap and joints that a - Paper box
Calculate the paper consumption on the box-shaped quadrangular prism with rhombic footstall, base edge a=6 cm, and the adjacent base edges form an angle alpha = 60 °. The box height is 10 cm. How much m² of the paper is consumed 100 such boxes?
