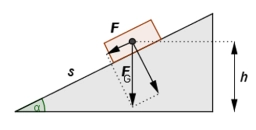
Tram - safe downhill
What is the maximum angle at which the tram can go downhill to still be able to stop? The coefficient of shear friction is f =0.15.
Final Answer:
Tips for related online calculators
See also our right triangle calculator.
See also our trigonometric triangle calculator.
Try conversion angle units angle degrees, minutes, seconds, radians, grads.
See also our trigonometric triangle calculator.
Try conversion angle units angle degrees, minutes, seconds, radians, grads.
You need to know the following knowledge to solve this word math problem:
planimetricsgoniometry and trigonometryUnits of physical quantitiesthemes, topicsGrade of the word problem
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Coefficient 45821
What is the smallest radius a turn must have for a car to enter safely without exceeding a speed of 50 km/h? The coefficient of shear friction between the tires and the surface is 0.4. - Shear friction
How much force must we apply to a box weighing 300 kg to move it uniformly along a horizontal floor if the coefficient of shear friction between the box's edge and the floor is 0.5? - Coefficient 70214
An 80 kg cart moving at constant speed along a horizontal road is subjected to a pulling force of 120 N. Specify: a) the magnitude of the frictional force b) the value of the coefficient of shear friction. - Friction coefficient
What is the weight of a car when it moves on a horizontal road at a speed of v = 50 km/h at engine power P = 7 kW? The friction coefficient is 0.07 - Coefficient of friction
A car moves along a horizontal road at a speed of 15 m/s. After turning off the engine, the car traveled a distance of 225 m. What was the coefficient of friction for this motion? - Ferko
Ferko boarded the tram, which already had 19 passengers. At the first stop, the tram left twice as few passengers, and at the second stop, twice less. The third stop was final. How many passengers left with Ferko at the third stop? - Coefficient 80515
A tractor pulls a tree trunk weighing 1.5 t for a distance of 2 km along a horizontal road at a constant speed. What mechanical work does it do if the coefficient of sliding friction is 0.6?
