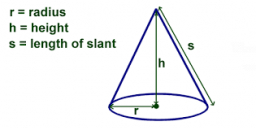
The radius
A right circular cone's radius and slant heights are 9 cm and 15 cm, respectively. Find, correct to one decimal place, the
(i) Height
(ii) Volume of the cone
(i) Height
(ii) Volume of the cone
Correct answer:
Tips for related online calculators
Need help calculating sum, simplifying, or multiplying fractions? Try our fraction calculator.
Tip: Our volume units converter will help you convert volume units.
See also our right triangle calculator.
Tip: Our volume units converter will help you convert volume units.
See also our right triangle calculator.
You need to know the following knowledge to solve this word math problem:
- algebra
- expression of a variable from the formula
- arithmetic
- square root
- solid geometry
- cone
- planimetrics
- Pythagorean theorem
- right triangle
- numbers
- fractions
Units of physical quantities:
Grade of the word problem:
Related math problems and questions:
- Slant height
Find the cone's volume. The cone's slant height is 5cm, and the radius of its base is 3cm. - The diagram 2
The diagram shows a cone with a slant height of 10.5cm. If the curved surface area of the cone is 115.5 cm². Calculate to correct three significant figures: *Base Radius *Height *Volume of the cone - Cone 15 The radius of the base of a right circular cone is 14 inches, and its height is 18 inches. What is the slant height?
- The diameter 4
The cone's diameter is 14ft, and the height is 7 ft. What is the slant height?
- Volume of cone
Find the volume of a right circular cone-shaped building with a height of 9 m and a radius base of 7 m. - Slant height 3
The frustum of a right circular cone has the diameters of base 10 cm of top 6 cm and a height of 5 cm. Find the slant height. - The base 2
The base diameter of a right cone is 16cm, and its slant height is 12cm. A. ) Find the perpendicular height of the cone to 1 decimal place. B. ) Find the volume of the cone, and convert it to 3 significant figures. Take pi =3.14
