Spherical cap
The spherical cap has a base radius of 8 cm and a height of 5 cm. Calculate the radius of a sphere of which this spherical cap is cut.
Correct answer:
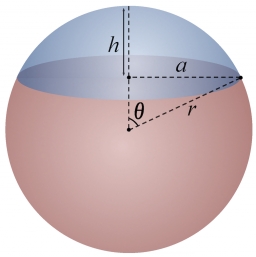
Tips for related online calculators
You need to know the following knowledge to solve this word math problem:
Related math problems and questions:
- Sphere - parts
Calculate the area of a spherical cap, which is part of an area with a base radius ρ = 10 cm and a height v = 3.4 cm. - Spherical cap
Place a part of the sphere on a 4.6 cm cylinder so that the surface of this section is 20 cm². Determine the radius r of the sphere from which we cut the spherical cap. - The roof
The roof has a spherical canopy with a base diameter of 8 m and a height of 2 m. Calculate the foil area with which the roof is covered when calculating 13% for waste and residues. - Hemisphere cut
Calculate the spherical layer's volume that remains from the hemisphere after the 3 cm section is cut. The height of the hemisphere is 10 cm.
- Spherical segment
The spherical segment with height h=2 has a volume of V=225. Calculate the radius of the sphere which is cut in this segment. - Calculate sphere cap
Calculate the surface of a spherical cap with a height of 6 cm and a radius of 15 cm - Sphere cut
A sphere segment is cut off from a sphere k with radius r = 1. The volume of the sphere inscribed in this segment is equal to 1/6 of the segment's volume. What is the distance of the cutting plane from the center of the sphere?
