Volume + expression of a variable from the formula - practice problems - page 4 of 33
Number of problems found: 649
- Calculate 81939
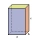The block surface is 5,632 m². The lengths of the edges are in the ratio 1: 2 : 3. Calculate the volume of the cuboid. - Calculate 81936 The volume of the block is 7,500 dm³. The lengths of the edges are in the ratio 3: 4: 5. Calculate the surface area of the cuboid.
- Calculate 81935 The volume of the cuboid is 960 cm³. The lengths of the edges are in the ratio 1 : 3: 5. Calculate the surface area of the cuboid.
- Dimensions 81850
We used the same amount of paint to paint a cuboid with dimensions of 10 cm, 15 cm, and 3 cm to paint the shell of a cone whose radius is 8 cm. How tall is this cone? Calculate its volume in liters.
- Calculate 81841
The sum of the lengths of all the cube's edges is 60 cm. How to calculate its volume? - Understanding 81807
The aquarium is 0.7m long and 25cm wide. The battery is deep if it can hold no more than 87.5 liters of water. I need help understanding how to calculate this. - Block-shaped 81632
The block-shaped pool is 40 meters long and 18 meters wide. Ten thousand eight hundred hectoliters of water were poured into it. How high is the water in it (how deep is the pool)? - Dimensions 81608
Find out if a circle with a volume of 38.5 cm² fits into a rectangle with dimensions of 110 mm and 65 mm. - Calculate 81560 The cone's surface is 75.36 cm, and the radius is 3 cm. Calculate the volume of the cone.
- Spherical 81527
Sketch a spherical layer formed from a sphere with a radius of r= 8.5cm, given: v=1.5cm, r1=7.7cm, r2=6.8cm. What is its volume? - Cylinder-shaped 81512
A truncated cone-shaped part with base radii of 4 cm and 22 cm is to be recast into a cylinder-shaped part of the same height as the original part. What base radius will the new part have? - Calculation 81401
A regular four-sided pyramid has a volume of 2,160 liters and a base edge length of 12 dm. Calculate the height of the needle (sketch, calculation, answer). - Revolution 81339
The rotating cone has a volume of 120 dm³. How tall is a cylinder of revolution with the same volume as a cone of revolution? - Determine 81311
The surface of the rotating cone and its base area is in the ratio 18:5. Determine the volume of the cone if its body height is 12 cm.
- Equilateral 81142
The rotating body was created by rotating an equilateral triangle with a side length of a=2 cm around one of its sides. Calculate the volume of this rotating body. - Toothpaste 81127
How long will the roll of toothpaste be extruded from the tube if the volume of the toothpaste is 100 ml and the diameter of the opening is 8 mm? - Calculate 81034
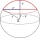Calculate the volume of the spherical segment and the surface area of the canopy if the radius of the sphere is r=5cm and the radius of the circular base of the segment ρ=4cm. - Quadrilateral 81033
The foundations of a regular truncated quadrilateral pyramid are squares. The lengths of the sides differ by 6 dm. Body height is 7 dm. The body volume is 1813 dm³. Calculate the lengths of the edges of both bases. - Circumscribed 81025
A cube with a volume of 4096 cm³ is described and inscribed by a sphere. Calculate how many times the volume of the circumscribed sphere is greater than the inscribed sphere.
Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
