Surface Area Calculation Problems for Solid Shapes. - page 24 of 50
Number of problems found: 993
- Church roof
The roof of the church tower has the shape of a regular tetrahedral pyramid with a base edge length of 5.4 meters and a height of 5 m. It was found that the 27% covering of the roof area needs to be corrected. What amount of material will be required? - Equilateral cylinder
The equilateral cylinder (height = base diameter; h = 2r) has a V = 272 cm³ volume. Calculate the surface area of the cylinder. - Tin with oil
Tin with oil has the shape of a rotating cylinder whose height is equal to the diameter of its base. The canned surface is 1884 cm². Calculate how many liters of oil are in the tin. - Cone
Calculate the volume and surface area of the cone with a diameter of the base d=16 cm and the side of the cone with the base has angle 37°12'.
- The cap
A rotating cone shapes a jester hat. Calculate how much paper is needed for the cap 53 cm high when the head circumference is 45 cm. - Calculation 83339
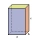The edges of a cuboid are in the ratio 1:2:3. Calculate their length if you know that the surface of the entire cuboid is S=5632 m². Then, perform a test to ensure the calculation is correct. - Decimeters 83242
The axial section of the cylinder is a square with an area of 56.25 cm². Calculate its surface area and volume. Express the result in square decimeters and cubic decimeters and round to hundredths. - Percentage 81914 The carpenter worked on a rotary cylinder with a base radius of 2.5 dm and a height of 2 dm. He reduced the radius by 1 cm by uniform grinding, and the height of the cylinder was preserved. Calculate the percentage by which the volume of the cylinder has
- Calculate 81034
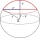Calculate the volume of the spherical segment and the surface area of the canopy if the radius of the sphere is r=5cm and the radius of the circular base of the segment ρ=4cm.
- Deviation 70434
Frustum has the base radii of the figures r1 and r2: r1> r2, r2 = s, and if the side deviation from the base plane is 60°. Express the surface and volume of the cone frustum using its side s. - Centimeters 65884
The plastic bucket has the shape of a cylinder with a diameter of 25 cm and a height of 40 cm. How many square centimeters are needed to produce it? How many liters does it contain when filled 5 cm below the rim? - Original 63974
If we reduce the length of the cube edge by 30%, this cube has a reduced surface area of 1176 cm². Find the edge length and volume of the original cube. - Calculate 26433
Calculate how much cm² of paper needs to be bought to make a 60 mm cube if you need to add an extra 12% to the folds. - Quadrilateral 15023
The regular quadrilateral pyramid has a base circumference of 44 cm and a body height of 3.2 cm. Calculate its volume and surface.
- Lampshade 7846
Lampshade for the face of a truncated cone with a height of 20 cm. The upper diameter of the shade is 13 cm, the lower 36 cm, and the side forms an angle of 60 degrees with the lower diameter. At least how much fabric is needed to make this shade? - Right-angled 6034
A three-sided prism has a base in the shape of a right-angled triangle with a length of 5 cm. The giant wall of the prism shell has a volume of 104 cm². The prism is 8 cm high. Calculate the volume and surface area of the prism. - Needed 5373
The box is shaped like a cube with an edge 52 cm long. How many m² of sheet metal is needed to make a box with a lid? Add 5% to the folds of the lid and walls. - Quadrilateral pyramid
The volume of a regular quadrilateral pyramid is 72 cm³. Its height is equal to the length of the base edge. Calculate the length of the base and the surface of the pyramid. - The perimeter
The perimeter of the base of a regular quadrilateral pyramid is the same as its height. The pyramid has a volume of 288 dm³. Calculate its surface area round the result to the whole dm².
The volume of a regular square prism is 192 cm³. The size of its base edge and the body height is 1:3. Calculate the surface of the prism.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
