Solid geometry, stereometry - page 111 of 117
Number of problems found: 2329
- The resistance
What is the resistance of an aluminum wire, 0.2 km long and 10 mm in diameter? - Cylinder-shaped 12761
During the experiment, water flows evenly into a cylinder-shaped container. At the end of the 5th minute of the experiment, the water level was 25% higher than at the beginning of the experiment. 0 how many percent higher will the water level be at the en - Horizontally 8187
We turn the prism-shaped box with a height of 1 m and a square base with an edge of 0.6 m under a force of 350 N, which acts horizontally compared to the upper edge. What is the weight of the box? - Prescribed 4579
The racing ball for men has a prescribed weight of 7250g. It is made of iron. How does its diameter change if we make it from lead? The density of iron is 7.8 g / cm cubic, and the density of lead is 11.3 t / m cubic.
- Fuel economy
How many kilometers is sufficient petrol in the cylinder fuel tank with a diameter of 40 cm and the base of tank length of 1 m when it is filled to 60%, and if the car consumes 15 liters per 100 km? - Equator 6020
The Equator. ..40075 km train. ..300m. How many trains would fit on the Equator? - Consecutive 46761
The block lengths are made up of three consecutive GP members. The sum of the lengths of all edges is 84 cm, and the volume block is 64 cm³. Determine the surface of the block. - Measuring cork
Simon boasted that he had taken away a block of cork measuring 0.5m x 0.5m x 1.2m. Is it possible we know that 1 m of cubic cork weighs 300 kg and children from 10 to 15 years old can carry a maximum load of 5 kg? - Freezer
The freezer has the shape of a cuboid with internal dimensions of 12 cm, 10 cm, and 30 cm. A layer of ice 23 mm thick was formed on the freezer's inner walls (and on the opening). How many liters of water will drain if we dispose of the freezer?
- Dice - 5 times
We roll the dice five times. Make sentences: a) 3 events that definitely cannot happen. Write a reason for each. b) 3 events that will definitely occur; write a reason for each. Another problem: 3 events that may or may not occur for each. Write a reason. - Semicircle 82687
If the shell of a cone is a semicircle, then the diameter of the cone's base is equal to its side's length. Prove it. - Quadrilateral 5277
Given a regular quadrilateral pyramid ABCDV, point M is inside its edge AV, and point N is on the long line DC beyond point C. Construct the intersection of the plane MNV with the plane BCV and the intersection of the line MN and the plane BCV. - Perpendicular 5865
We cut the cube with two mutually perpendicular cuts, each parallel to one of the cube's walls. By what percentage is the sum of the surfaces of all cuboids created in this way greater than the surface of the original cube? - Distribute 32451
The king cannot decide how to distribute 4 cubes of pure gold, which have edges of length 3cm, 4cm, 5cm, and 6cm, to two sons as fairly as possible. Design a solution so that the cubes do not have to be cut.
- Average speed
What is the average speed you have to move around the world in 80 days? (Path along the equator, round to km/h). - Gold wire
From one gram of gold was pulled wire 1.4 km length. What is its diameter if the density of Au is ρ=19.5 g/cm³? - Megapascals
What is the area of cross-section of the piston if the force of 300 kN produces a pressure of 5 MPa? - Perpendicular 3494
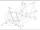In axonometry, construct the projection of a perpendicular 4-sided pyramid with a square base ABCD in the plane. The base triangle gives the axonometry. We know the center of the base S, the point of the base A, and the height of the pyramid v. - Pillar
Calculate the volume of the pillar shape of a regular tetrahedral truncated pyramid if his square has sides a = 10, b = 19, and height is h = 28.
Sketch a grid of a quadrilateral prism with a rectangle of 1 cm x 3 cm and a height of 5 cm.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
