Quadratic equation + square (second power, quadratic) - practice problems - page 13 of 15
Number of problems found: 292
- BMI index
Calculate BMI (body mass index, an index indicating obesity, overweight, normal weight, underweight) man weighing m = 102 kg and height h = 159 cm. The index is calculated according to the equation (formula): BMI = (m)/(h²) With the BMI index, it is possi - The hall
The hall had a rectangular ground plan, one dimension 20 m longer than the other. After rebuilding, the length of the hall declined by 5 m, and the width increased by 10 m. The floor area increased by 300 m². What were the original dimensions of the hall? - Orchard
The route passes the trapezoidal orchard perpendicular to the parallel sides. It is 80 cm wide. The lengths of the bases are in the ratio 5:3. The length of the longer base to the size of the path is in the ratio of 5:6. How many square meters occupy the - Perimeter and legs
Determine the perimeter of a right triangle if the length of one leg is 75%, the length of the second leg, and its area is 24 cm².
- 3d vector component
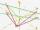The vector u = (3.9, u3), and the length of the vector u is 12. What is, is u3? - Cylinder diameter
The surface of the cylinder is 112 cm². The cylinder height is 5 cm. What is the diameter of this cylinder? - QuizQ2
The square of the first number is equal to three-fifths of the second number. Determine both numbers if you know that the second number is five times greater than the first, and neither of the numbers is not equal to zero. - Quadratic function
It is given a quadratic function y = -4x²+5x+c with an unknown coefficient c. Determine the smallest integer c for which the graph of f intersects the x-axis at two different points. - Diagonals in the diamond
The length of one diagonal in a diamond is 24 cm greater than the length of the second diagonal, and the diamond area is 50 m². Determine the sizes of the diagonals.
- Tiles
From how many tiles, 20 cm by 30 cm, we can build a square of maximum dimensions if we have a maximum of 275 tiles. - Circle
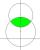The circle is given by the center on S[-7; 10], and the maximum chord is 13 long. How many intersections have a circle with the coordinate axes? - Abyss
The stone fell into the abyss: 11 seconds after we heard it hit bottom. How deep is the abyss (neglecting air resistance)? (gravitational acceleration g = 9.81 m/s² and the speed of sound in air v = 336 m/s) - Equation
Equation -2x²+bx -82 =0 has one root x1 = -8. Determine the coefficient b and the second root x2. - Rectangle - sides
A rectangle has an area 340 cm². The length of the shorter side is 3 cm fewer than the length of the longer side. What is the perimeter of a rectangle?
- Rhombus
The rhombus with area 95 has one diagonal that is longer by 7 than the second one. Calculate the length of the diagonals and rhombus sides. - Built-up area
John build-up area 4.3 x 6.3 = 27.09 m² with building with a wall thickness 25 cm. How many centimeters would he have to subtract from the thickness of the walls that the built-up area fell by 5%? - Tank
In the middle of a cylindrical tank with a bottom diameter of 479 cm, there is a standing rod 34 cm above the water surface. If we bank the rod, its end reaches the water's surface just by the tank wall. How deep is the tank? - Pure quadratic equation
Solve pure quadratic equation -7x² +4 = 0. - Rhombus and inscribed circle
It is given a rhombus with side a = 6 cm and the inscribed circle r = 2 cm radius. Calculate the length of its two diagonals.
If to a square of integer number add 43, we get the square of the following integer number. What is the original number?Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
