Quadratic Equations Problems - page 11 of 30
Number of problems found: 583
- Intersections 3
Find the intersections of the circles x² + y² + 6 x - 10 y + 9 = 0 and x² + y² + 18 x + 4 y + 21 = 0 - On a line
On a line p : 3 x - 4 y - 3 = 0, determine the point C equidistant from points A[4, 4] and B[7, 1]. - Shell area cy
The cylinder's shell area is 300 cm square, and its height is 12 cm. Calculate its volume. - The cylinder
The cylinder's surface area is 300 square meters, and its height is 12 meters. Calculate its volume.
- Function 3
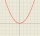Function f(x)=a(x-r)(x-s) the graph of the function has an x-intercept at (-4, 0) and (2, 0) and passes through the point (-2,-8). Find constant a, r, s. - Variations 26791
If the number of elements increases by two, the number of variations of the second class of these elements created by 38 increases. What is the original number of elements? - Circumference 26651
A rectangle with sides of lengths a, b (cm) has a circumference of 100 cm. The dependence of its area P (in cm2) on the number a can be expressed by the quadratic function P = sa + ta². Find the coefficients s, t. - Rectangular 26641
The area of the work surface of the rectangular table is 70 dm2, and its perimeter is 34 dm. Determine (in dm) the length of the shorter side of this table. - Lookout tower
How high is the lookout tower? If each step was 3 cm lower, 60 more were on the lookout tower. If it were 3 cm higher again, it would be 40 less than it is now.
- 1 page
One page is torn from the book. The sum of the page numbers of all the remaining pages is 15,000. What numbers did the pages have on the page that was torn from the book? - Two chords
From the point on the circle with a diameter of 8 cm, two identical chords are led, which form an angle of 60°. Calculate the length of these chords. - Determine 25341
In a two-digit number, the number of tens is three more than the number of ones. If we multiply the original number by a number written with the same digits but in the reverse order, we get the product 3 478. Determine the actual number. - Intersections 25141 The quadratic function has the formula y = x²-2x-3. Sketch a graph of this function. Find the intersections with the axes. Find the vertex coordinates.
- Calculate 25111
The quadratic function has the formula y = -2x²-3x + 8. Calculate the function value in points 5, -2, and ½.
- Circle and square
An ABCD square with a side length of 100 mm is given. Calculate the circle’s radius that passes through vertices B, C, and the center of the side AD. - Magnified cube
If the lengths of the cube's edges are extended by 5 cm, its volume will increase by 485 cm³. Determine the surface of both the original and the magnified cube. - Viewing angle
The observer sees a straight fence 60 m long at a viewing angle of 30°. It is 102 m away from one end of the enclosure. How far is the observer from the other end of the enclosure? - A map
A map with a scale of 1:5,000 shows a rectangular field with an area of 18 ha. The length of the field is three times its width. The area of the field on the map is 72 cm square. What is the actual length and width of the field? - Birthdays
In the classroom, students always give candy to their classmates on their birthdays. The birthday person always gives each one candy, and he does not give it himself. A total of 650 candies were distributed in the class per year. How many students are in
Solve the quadratic equation: 2y²-8y + 12 = 0Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
