Center of gravity and median
In the isosceles triangle ABC, the center of gravity T is 2 cm from the base AB. The median parallel to the AB side measures 4 cm. What is the area of the ABC triangle?
Correct answer:
Tips for related online calculators
Check out our ratio calculator.
See also our right triangle calculator.
Calculation of an isosceles triangle.
See also our trigonometric triangle calculator.
See also our right triangle calculator.
Calculation of an isosceles triangle.
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Perimeter 6002
In the triangle ABC, there is a side c = 5cm and medians ta = 6cm (median to side a), tb = 4.5cm (median to side b). Find the perimeter of the triangle ABT (T = center of gravity). - Medians 2:1
The Median to side b (tb) in triangle ABC is 12 cm long. a. What is the distance of the center of gravity T from vertex B? b, Find the distance between T and the side b. - Center of gravity
In the isosceles triangle ABC the lengths of AB and the height to AB is the ratio of 10:12. The arm has a length of 26 cm. If the center of gravity is T, find the area of the triangle ABT. - Calculate 4425
In the triangle ABC with the center of gravity T, b = 7cm, median to c: tc = 9cm, the ATC angle is 112 degrees. Calculate the length of the line ta.
- Isosceles 5575
The picture shows an isosceles triangle VLK with a center of gravity of T. The base VL measures 16 cm, and the line KK1 measures 18 cm. How long is the VV1 line? - Construction
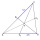Construct the triangle ABC if you know: the size of the side AC is 6 cm, the size of the angle ACB is 60°, and the distance of the center of gravity T from the vertex A is 4 cm. (Sketch, analysis, notation of construction, construction) - Ratio of triangles areas
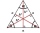In an equilateral triangle ABC, the point T is its center of gravity, the point R is the image of the point T in axial symmetry along the line AB, and the point N is the image of the point T in axial symmetry along the line BC. Find the ratio of the areas
