A plane vs. sphere
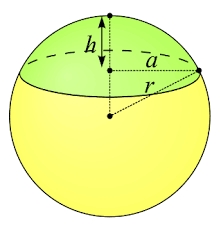
The intersection of a plane is 2 cm from the sphere's center, and this sphere is a circle whose radius is 6 cm. Calculate the surface area and volume of the sphere.
Correct answer:
Tips for related online calculators
Need help calculating sum, simplifying, or multiplying fractions? Try our fraction calculator.
See also our right triangle calculator.
Tip: Our volume units converter will help you convert volume units.
See also our right triangle calculator.
Tip: Our volume units converter will help you convert volume units.
You need to know the following knowledge to solve this word math problem:
- algebra
- expression of a variable from the formula
- arithmetic
- square root
- solid geometry
- sphere
- surface area
- spherical cap
- planimetrics
- Pythagorean theorem
- right triangle
- area of a shape
- numbers
- fractions
Units of physical quantities:
Grade of the word problem:
Related math problems and questions:
- Sphere
Intersect between the plane and a sphere is a circle with a radius of 60 mm. The cone, whose base is this circle and whose apex is at the center of the sphere, has a height of 34 mm. Calculate the surface area and volume of a sphere. - Surface and volume
Calculate the surface and volume of a cylinder whose height is 8 dm and the radius of the base circle is 2 dm. - Calculate 24881
Calculate a sphere's volume and surface area with a radius of 23dm. - Spheres in sphere
How many spheres with a radius of 15 cm can fit into the larger sphere with a radius of 150 cm?
- Sphere cuts
At what distance from the center does the sphere intersect with the radius R = 91 plane if the cut area and area of the main sphere circle are in ratio 3/6? - Sphere cut
A sphere segment is cut off from a sphere k with radius r = 1. The volume of the sphere inscribed in this segment is equal to 1/6 of the segment's volume. What is the distance of the cutting plane from the center of the sphere? - A spherical segment
The aspherical section, whose axial section has an angle of j = 120° in the center of the sphere, is part of a sphere with a radius r = 10 cm. Calculate the cut surface.
