Statements true/false
Tips for related online calculators
You need to know the following knowledge to solve this word math problem:
Grade of the word problem:
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- Triangular pyramid
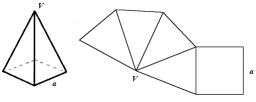
A regular tetrahedron is a triangular pyramid whose base and walls are identical equilateral triangles. Calculate the height of this body if the edge length is a = 8 cm. - Equilateral 83322
The glass weight has the shape of a regular four-sided pyramid with a base edge of 10 cm. The shell walls are equilateral triangles. What is the weight in grams of the paperweight if the density of the glass is 2500kg/m³? - 40cmx60cm 83619
The stand on which the posters are stuck has the shape of a cone. It is 2.4m tall. The side of the cone is 2.5 m long. How many 40cmx60cm posters can be stuck on the stand so they do not overlap? - Maximum of volume
The shell of the cone is formed by winding a circular section with a radius of 1. For what central angle of a given circular section will the volume of the resulting cone be maximum?
- Right-angled trapezoid
A right-angled trapezoid with the measure of the acute angle of 50° is given. The lengths of its bases are 4 and 6 units. The volume of the solid obtained by rotation of the given trapezoid about the longer base is: - Equilateral cone
We pour so much water into a container with the shape of an equilateral cone, the base of which has a radius r = 6 cm, that one-third of the volume of the cone is filled. How high will the water reach if we turn the cone upside down? - Surface of the cylinder
Calculate the surface of the cylinder for which the shell area is Spl = 20 cm² and the height v = 3.5 cm.
