Sine - practice problems - page 2 of 16
Number of problems found: 306
- Observatories A,B
The target C is observed from two artillery observatories, A and B, 296 m apart. At the same time, angle BAC = 52°42" and angle ABC = 44°56". Calculate the distance of the target C from observatory A. - Parallelogram 82695
Given is the parallelogram KLMN, in which we know the side sizes/KL/ = a = 84.5 cm, /KN/ = 47.8 cm, and the angle size at the vertex K 56°40'. Calculate the size of the diagonals. - Circular 82418
A circular segment has an area of 6.04 cm², the central omega angle is 15 degrees, what is the radius? - Cone slope
Determine the volume and surface area of a cone whose slope of length 8 cm makes an angle of 75 degrees with the plane of the base.
- Calculate 81950
The tangent of the angle formed by the adjacent sides of the triangle ABC (side a=29 m, b = 40 m) equals 1.05. Calculate the area of that triangle. - Difference 81888
The ropeway climbs at an angle of 22°30'. Calculate its length if the height difference between the lower and upper station is 560m. Sketch a picture - Perpendicular 81758
Distribute the force of magnitude F = 100 N into two perpendicular components with magnitudes F1 and F2 so that the angle between forces F1 and F is 43°52'. - Archaeologists 81478
Archaeologists need to find out the size of the vessel if the sherd found was in the shape of a circular section with a length of 12 cm and a height of 3 cm. What is the area of this section? - Quadrilateral 81097
The quadrilateral ABCD is symmetrical about the diagonal AC. The length of AC is 12 cm, the length of BC is 6 cm, and the interior angle at vertex B is right. points E and F are given on the sides AB, and AD so that the triangle ECF is equilateral. Determ
- Circle's 81078
The chord of a circle is 233 long, and the length of the circular arc above the chord is 235. What is the circle's radius, and what is the central angle of the circular arc? - In the desert
A man wondering in the desert walks 5.7 miles in the direction S 26° W. He then turns 90° and walks 9 miles in the direction N 49° W. At that time, how far is he from his starting point, and what is his bearing from his starting point? - Felix
Calculate how much land Felix Baumgartner saw after jumping from 36 km above the ground. The radius of the Earth is R = 6378 km. - Crosswind
A plane is traveling 45 degrees N of E at 320 km/h when it comes across a current from S of E at 115 degrees of 20 km/h. What are the airplane's new course and speed? - Acceleration 79164
A skier goes down a slope 66 m long in a uniformly accelerated motion in 10 seconds. With what acceleration was it moving, and what is the slope of the slope?
- Instantaneous 76754
For a dipole, calculate the complex apparent power S and the instantaneous value of the current i(t), given: R=10 Ω, C=100uF, f=50 Hz, u(t)= square root of 2, sin( ωt - 30 °). Thanks for any help or advice. - The cosine law
Solve the unknown dimensions for the following triangle: Triangle ABC: Angle A=43 degrees, b=7.0cm, c=6.0cm Question 1. Angle B with units written as degrees Question 2. Angle C with units written as degrees Question 3. a, rounded to the nearest tenth of - Sin cos tan
If cos y = 0.8, 0° ≤ y ≤ 90°, find the value of (4 tan y) / (cos y-sin y) - A ship
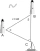A ship has been spotted by two lighthouses, A and B, as shown in the figure. What is the distance from the ship to Lighthouse A to the nearest tenth? Figure - the distance between lighthouses A and B is 40 nautical miles. From A is seen in view angle 57° - A Ferris wheel
A Ferris wheel with a diameter of 100 feet makes five revolutions every 8 minutes. The base of the wheel is 4 feet above the ground. Your friend gets on at 3 PM sharp. a) Write an equation in seconds to express your friend's height in feet at any given ti
Triangle ABC has angle C bisected and intersected AB at D. Angle A measures 20 degrees, and angle B measures 40 degrees. The question is to determine AB-AC if length AD=1.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
