Similarity of triangles - practice problems - page 5 of 8
Number of problems found: 153
- The chimney
The chimney casts a shadow 45 meters long. The one-meter-long rod standing perpendicular to the ground has a shadow 90 cm long. Calculate the height of the chimney. - Lighthouse
Marcel (point J) lies in the grass and sees the top of the tent (point T) and, behind it, the top of the lighthouse (P). | TT '| = 1.2m, | PP '| = 36m, | JT '| = 5m. Marcel lies 15 meters away from the sea (M). Calculate the lighthouse distance from the s - Trapezium diagonals
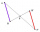It is given trapezium ABCD with bases | AB | = 12 cm, |CD| = 8 cm. Point S is the intersection of the diagonals for which |AS| is 6 cm long. Calculate the length of the full diagonal AC. - Diagonals at right angle
In the trapezoid ABCD, this is given: AB=12cm CD=4cm And diagonals crossed under a right angle. What is the area of this trapezoid ABCD?
- Mast shadow
The mast has a 13 m long shadow on a slope rising from the mast foot toward the shadow angle at an angle of 15°. Determine the height of the mast if the sun above the horizon is at an angle of 33°. Use the law of sines. - Shadow 7838
A man 1.65 m tall casts a shadow of 1.25 m. How tall is the tree whose shadow is in debt 2.58 m? - Isosceles 7661
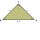The area of the isosceles triangle is 8 cm2, and its arm's length is 4 cm. Calculate the sizes of its interior angles. - Rectangular trapezoid
The ABCD rectangular trapezoid with the AB and CD bases is divided by the diagonal AC into two equilateral rectangular triangles. The length of the diagonal AC is 62cm. Calculate the trapezium area in cm square and calculate how many different perimeters - Calculate 7214
Two tangents are drawn from point C to a circle with a radius of 76 mm. The distance between the two contact points is 14 mm. Calculate the distance of point C from the center of the circle.
- Equilateral triangle ABC
In the equilateral triangle ABC, K is the center of the AB side, the L point lies on one-third of the BC side near point C, and point M lies on one-third of the side of the AC side closer to point A. Find what part of the ABC triangle contains the triangl - Pyramid cut
We cut the regular square pyramid with a parallel plane to the two parts (see figure). The volume of the smaller pyramid is 20% of the volume of the original one. The bottom of the base of the smaller pyramid has an area of 10 cm². Find the area of the or - Shadow A meter pole perpendicular to the ground throws a shadow of 40 cm long. The house throws a shadow 6 meters long. What is the height of the house?
- Triangles 6682
Triangles ABC and A'B'C'. They are similar. In triangle ABC, the measures of the two angles are 25 degrees and 65 degrees. Explain why, in triangle A'B'C', the sum of the sizes of the two angles is equal to 90 degrees. - Isosceles 6673
Isosceles triangle X'Y'Z' . It is similar to triangle XYZ. The base of triangle XYZ has length |XY|=4cm. The size of the angle at the X vertex is 45 degrees. Draw a triangle X'Y'Z' whose base is 8 cm long.
- Coefficient 6672
In the triangle ABC is [AB] = 20cm, [BC] = 10cm, A = 30 °. Construct a triangle A'B'C' similar to triangle ABC if the similarity coefficient is 0.5 - Following 6660
KLM triangle on sides k = 5.4 cm. L = 6 cm, m = 6.6 cm. Construct a triangle K 'L'M 'for which the following holds: ∆KLM ~ ∆K 'L'M' and m '= 9.9cm - Intersection 6653
Two straight paths cross, making an angle alpha = 53 degrees 30'. There are two pillars on one of them, one at the intersection, the other at a distance of 500m from it. How far does one have to go from the intersection along the other road to see both po - Triangles 6647 For triangles ABC and A'B'C': alpha = alpha with a line, beta with line = beta. a) are these triangles identical? Why? b) are these triangles similar? Why?
- Two angles
The triangles ABC and A'B'C 'are similar. In the ABC triangle, the two angles are 25° and 65°. Explain why in the triangle A'B'C 'is the sum of two angles of 90 degrees.
Find the area of an isosceles trapezoid if the lengths of its bases are 16 cm and 30 cm and the diagonals are perpendicular to each other.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
