Sets - practice problems - page 9 of 16
Number of problems found: 303
- Intersections 26781
A rectangular grid consists of two mutually perpendicular systems of parallel lines with a distance of 2. We throw a circle with a diameter of 1 on this plane. Calculate the probability that this circle: a) overlaps one of the straight lines; b) do any of - Coordinates
Determine the coordinates of the vertices and the area of the parallelogram, the two sides of which lie on the lines 8x + 3y + 1 = 0, 2x + y-1 = 0, and the diagonal on the line 3x + 2y + 3 = 0 - Two-digit 26211
Write all two-digit numbers using the numbers 4 and 7 - Probability
In a batch of 500 products, there are 10 rejects. In a statistical inspection, 40 products are checked, which are randomly selected from a batch, and the selection is made without repetition. The batch is considered satisfactory if there is at most one de - Statistically 25181
Statistically, in a city of 100,000 inhabitants, 600 people die in one year. During the year, 2,000 people received hospital treatment, and 120 of them died there. Calculate the probability that a citizen who comes to the hospital for treatment will die t - Cutting the prism
A prism with a square base with an area of 1 cm² and a height of 3 cm was cut from a cube with an edge length of 3 cm. What is the body's surface formed from the cube after cutting the prism? - Defective 23221
The factory sets a standard that 2% of products may be defective. Last week, out of 1,250 products, 32 were defective. How many defective products were the standard exceeded?
- T-shirts 22133
There were 40 boys in the school. They were branded with caps and t-shirts. Twenty-five boys had blue caps. Twenty boys had a blue cap and a yellow T-shirt. a) How many boys had a yellow shirt? b) How many boys did not have a blue cap? c) How many boys on - Distance 22043
There is a given circle k (S, 4 cm) and a line p. If the distance of point S from line p is less than 4 cm, is the line p called? - Double probability
The probability of success of the planned action is 60%. What is the probability that we will achieve success at least once if this action is repeated twice? - Individual 21713
They bought 22 sets of crayons for the school group. Larger sets cost CZK 65, and smaller ones cost only CZK 50. They paid CZK 1,280 for all sets. How many individual types of crayon sets did they buy? - Alarm systems
What is the probability that at least one alarm system will signal the theft of a motor vehicle when the efficiency of the first system is 90% and of the independent second system 80%? - Butterflies and bees
There were 35 flowers in the garden. The butterflies were on 17 flowers. There were butterflies and bees on eight flowers. Can you find out how many flowers were only bees? Note. There was a maximum of one butterfly and a maximum of one bee on each flower - Fall sum or same Find the probability that if you roll two dice, the sum of 10 will fall, or the same number will fall on both dice.
- The university
At a certain university, 25% of students are in the business faculty. Of the students in the business faculty, 66% are males. However, only 52% of all students at the university are male. a. What is the probability that a student selected at random in the
- Median or middle
The number of hours of television watched per day by a sample of 28 people is given below: 4, 1, 5, 5, 2, 5, 4, 4, 2, 3, 6, 8, 3, 5, 2, 0, 3, 5, 9, 4, 5, 2, 1, 3, 4, 7, 2, 9 What is the median value? - Distance 15203
In the plane, the points A, B, and C are given 3 cm apart, and they do not lie in the same straight line. Mark the set of all points whose distance from all three points is less than or equal to 2.5 cm. - Return 15193
Fiona sets off from the tower after Shrek at 9:10 at a speed of 6 km/h. Shrek starts to meet her from the swamp at 9:50 at a speed of 4 km/h. At 10:50, they meet and return back to the swamp at a speed of 4 km/h. What time does he arrive at the swamp? - Christmas or Easter
Please calculate this example by the Venn equation. They asked 73 students whether they liked Christmas or Easter. Thirty-four of them like one of the holidays. 39 loves Easter. There are twice as many students who wish for both holidays as those who only - Eq triangle minus arcs
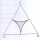In an equilateral triangle with a 2cm long side, the arcs of three circles are drawn from the centers at the vertices and radii 1cm. Calculate the area of the shaded part - a formation that makes up the difference between the triangle area and circular cu
Do you have unsolved problem that you need help? Ask a question, and we will try to solve it. Solving math problems.
