Sets - practice problems - page 7 of 16

Number of problems found: 304

Three subjects
In a class of 40 students, 18 passed mathematics, 19 passed accounting, 16 passed economics, five mathematics and accounting only, six mathematics only, nine accounting only, and two accounting and economics only. Each student was offered at least one of
Combinations
Evaluate the following expression involving combinations and permutations: C(6,3) + 3 P(6,3)
A swimmer-training
A swimmer-training program has 26 students specializing in butterfly stroke, 24 specializing in breaststroke, and 29 specializing in backstroke. Of these students, five specialize in all three strokes, five specialize in only the breaststroke and the back
25 pupils
Twenty-five pupils attend the school dance group, which is 5 percent of all pupils. The judo class is attended by 20 pupils, a quarter of whom also attend a dance group. How many schoolchildren do not go to a dance or judo group?
Gym center
80% of all visitors to the gym center enjoy a discount. 3/4 of all visitors go to practice regularly. All visitors who go to the gym regularly benefit from a discount. What percentage of all visitors do not go to the gym regularly but still use the discou
Coloured teacups
The teacups in Tea Stop 55 are `2/5` green and `3/10` yellow. What fraction of the teacups are neither green nor yellow?
The collector
Harry liked to collect old movies. On four different days, he bought two movies. On two other days, he purchased three movies each day. After that, he sold two sets of 2 movies. He figured out how many he had in total, and then he divided that total into
They bought
They bought the students a set of notebooks for 65 euros. The first set cost € 2 and the second € 3. There were five times as many two-euro as three-euro sets. How many sets of workbooks are there?
Solutions 41771
There were 32 toys on the toy store shelf. We know that there were 19 cars with these toys. We also know that 18 of these toys were made of wood. How many wooden cars were on the shelf? Find at least two solutions.
Divisors 40571
All common divisors of headings 90 and 48
Calculate 39131
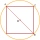A circle describes a square with a side of 8 cm. Calculate the area of the rest of the circle if we cut out the square.
Four children
What is the probability that in a family with four children, there are: a) at least three girls b) at least one boy, If the probability of a boy is 0.51?
Simultaneously 37091
Ten children came to the art circle. Eight children painted, and nine, I guess. How many children did I paint with, and I think simultaneously?
Distinguished 37083
Three circles of the same size are drawn on the playing field. Arrange the 16 pins so that there are 9 pins in each circle. Find at least eight significantly different layouts, i.e. J. such layouts in which pins or circles are not distinguished.
Goalkeeper
Mike plays as the goalkeeper 50% of the time if Peter is the coach in a football game. However, if Robert were coaching, he would only have a 30% chance. Peter coaches more than Robert in about 6 to 10 games. What is the probability that Mike gets to be t
Group of children
There is a group of children. There is a boy named Adam in each of the three children subgroups and a girl named Beata in each quartet (four-member subgroup). How many children can be in such a group, and what are their names in that case?
Open intervals
Open intervals A = (x-2; 2x-1) and B = (3x-4; 4) are given. Find the largest real number for which A ⊂ B applies.
Operations with sets
Set B - A has twice as few elements as set A - B and four times fewer elements than set A ∩ B. How many times more elements does set A have than set B?
MF graduate
78 school students graduate in mathematics or physics. Three times more students graduate from mathematics and do not graduate from physics than those who graduate from physics and do not graduate from mathematics. 69 students graduate in mathematics. How
Subtracting sets
For two sets K, L is true: K has 30 elements, L has 27 elements, and the set L - K has 22 elements. How many elements does the set K - L have?

Do you have unsolved math question and you need help? Ask a question, and we will try to solve it. We solve math question.