Multiplication + reason - practice problems - page 16 of 18
Number of problems found: 360
- Hockey match
The hockey match ended with a result of 3:1. How many different storylines may the match have? - Pennies
Eight hundred pennies have the same value as 100 ducats. One hundred pennies have the same value as 250 tolars. How many ducats have the same value as 100 tolars? - A family
A family of four pays for garbage collection per the year 1800 CZK. How many CZK (Czech crowns) a year does the waste company get from the house where live 93 people live? - The confectionery
The confectionery sold five kinds of ice cream. If the order of ice cream does not matter, how many ways can I buy three kinds?
- Students
The class has 22 students, 2 of whom have assessments of 2. The number of students with an assessment of 1 is three times more than the number of students with an assessment of 3. How many students have an assessment of 1, and how many students have an as - Athletes
Athletes are standing in rows of eight. Ivan estimated that there are more than 120, while Peter claimed that there are fewer than 130. Both are right. How many are athletes? - Difference 1632
The difference between the two numbers is 7. Their product 144. What are the numbers? - Grower
Grower harvested 160 kg of apples. Pears harvested ten times less. a) How many kilograms of pears were harvested? b) How many apples and pears were harvested? c) How many kg harvested pears less than apples? - The horses
A couple of horses consume 88 kg of oats for 14 days. How many oats were consumed by seven horses at the same consumption for six days?
- The camp
At the end of the camp, a 8 friends exchanged addresses. Any friend gave their remaining 7 friends their card. How many addresses did they exchange? - Three digits number
From the numbers 1, 2, 3, 4, and 5, create three-digit numbers whose digits do not repeat, and the number is divisible by 2. How many numbers are there? - Three digits number
How many are three-digit integers such that they have no digit repeats? - Chess
How many different ways can you initiate a game of chess (first pass)? - Wood bars
It is 9 bars 14 meters long and 9 bars 11 meters long. How many 5-meter laths are needed to make them?
- Seven-segmet
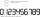Lenka is amused that he punched a calculator (seven-segment display) number and used only digits 2 to 9. Some numbers have the property that She again gave their image in the axial or central symmetry some number. Determine the maximum number of three-dig - Snowman 2
On the medal, which has the shape of a circle with a diameter 18 cm, is sketched a snowman so that the following requirements are met: 1. snowman is composed of three circles, 2. space over the snowman is the same as under it, 3. diameters of all circles - The largest number
Find the largest integer such that: 1. No figures are not repeated, 2. The multiplication of every two digits is odd, 3. In addition, all digits are odd. - Training
The table contains the tennis training schedule for Saturday's younger students during the winter indoor season. Before the start of the summer season, preparing a new training schedule, Tomas Kucera will be able to practice only in the morning. Sisters K - Snowman
In a circle with a diameter of 40 cm are drawn three circles (as a snowman) where: its diameters are integers, each larger circle diameter is 2 cm larger than the diameter of the previous circle. Determine the snowman height if we wish for the highest sno
Letter game Logik is a two-player game that has the following rules: 1. The first player thinks five-letter word in which no letter is not repeated. 2. The second player writes a five-letter word. 3. The first player answers two numbers. The first number Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
