Length + similarity of triangles - practice problems - page 3 of 4
Number of problems found: 63
- Isosceles 7661
The area of the isosceles triangle is 8 cm2, and its arm's length is 4 cm. Calculate the sizes of its interior angles. - Rectangular trapezoid
The ABCD rectangular trapezoid with the AB and CD bases is divided by the diagonal AC into two equilateral rectangular triangles. The length of the diagonal AC is 62cm. Calculate the trapezium area in cm square and calculate how many different perimeters - Isosceles 6673
Isosceles triangle X'Y'Z' . It is similar to triangle XYZ. The base of triangle XYZ has length |XY|=4cm. The size of the angle at the X vertex is 45 degrees. Draw a triangle X'Y'Z' whose base is 8 cm long. - Area of iso-trap
Find the area of an isosceles trapezoid if the lengths of its bases are 16 cm and 30 cm and the diagonals are perpendicular to each other.
- Concerning 6294
Two isosceles triangles have the same angle at the apex concerning the base. One has a 17 cm long arm and a 10 cm long base. The second has a base length of 8 cm. Determine the length of his arm. - A boy
A boy of 1.7m in height is standing 30m away from the flagstaff on the same level ground. He observes that the angle of deviation of the top of the flagstaff is 30 degrees. Calculate the height of the flagstaff. - Shadow of tree
Miro stands under a tree and watches its shadow and shadow of the tree. Miro is 180 cm tall, and its shade is 1.5 m long. The tree's shadow is three times as long as Miro's shadow. How tall is the tree in meters? - Calculate 5148
At a distance of 10 m from the river bank, they measured the base AB = 50 m parallel to the bank. Point C on the other bank of the river is visible from point A at an angle of 32°30' and from point B at an angle of 42°15'. Calculate the width of the river - Mirror
How far must Paul place a mirror to see the top of the tower 12 m high? The height of Paul's eyes above the horizontal plane is 160 cm, and Paul is from the tower distance of 20 m.
- Display case
Place a glass shelf at the height of 1m from the bottom of the display case in the cabinet. How long platter will we place at this height? The display case is a rectangular triangle with 2 m and 2.5 m legs. - Thales
Thales is 1 m from the hole. The eyes are 150 cm above the ground and look into the hole with a diameter of 120 cm, as shown. Calculate the depth of the hole. - Hexagon cut pyramid
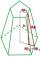Calculate the volume of a regular 6-sided cut pyramid if the bottom edge is 30 cm, the top edge is 12 cm, and the side edge length is 41 cm. - Sides of the triangle
The sides of the triangle ABC have a length of 4 cm, 5 cm, and 7 cm. Construct triangle A'B'C', similar to triangle ABC, which has a circumference of 12 cm. - V-belt
Calculate the length of the V-belt when the diameter of the pulleys is: D1 = 600 mm D2 = 120 mm d = 480 mm (distance between pulley axes)
- Sun rays
If the sun's rays are at an angle of 60°, then the famous Great Pyramid of Egypt (which is now 137.3 meters high) has a 79.3 m long shadow. Calculate the current height of the neighboring Chephren pyramid, whose shadow is measured at the same time at 78.8 - Ruler
Peter is looking at John over a ruler that keeps at an arm's distance of 60 cm from the eye, and on the ruler, John measured the height of 15 mm. John is 2 meters high. How far from Peter stands John? - Tree shadow
The tree perpendicular to the horizontal surface has a shadow 8.32 meters long. At the same time, a one-meter rod perpendicular to the horizontal surface has a shadow 64 cm long. How tall is the tree? - Catheti
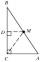One of the catheti of the right triangle has a length of 12 cm. At what distance from the center of the hypotenuse is another cathetus? - TV diagonal
A diagonal TV is 0.56 m long. How big is the television screen if the aspect ratio is 16:9?
The similarity ratio of two equilateral triangles is 4.3 (i.e., 43:10). The length of the side of the smaller triangle is 7.5 cm. Calculate the perimeter and area of the larger triangle.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
