Angle + similarity of triangles - practice problems
Number of problems found: 63
- The angles 6
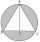If the angles of a triangle are in the ratio 2 : 3: 4. Find the value of each angle. - Traffic laws
Under traffic regulations, car lights can illuminate the road up to a maximum of 30 m. To check the reach of their car's dipped-beam lights, Peter stopped the car 1.5 m from the wall. The dipped-beam headlights are 60 cm high. At what height on the wall d - Intersection 6653
Two straight paths cross, making an angle alpha = 53 degrees 30'. There are two pillars on one of them, one at the intersection, the other at a distance of 500m from it. How far does one have to go from the intersection along the other road to see both po - Approximately 25381
The observer sees the tops of two trees at the same angle a. It is 9 m from one tree and 21 m from the other. The trees stand on a level. How tall is the second tree if the height of the first is 6 m? Remember that the eyes of a standing person are approx
- Distance 11711
The observer sits in a room 2 m from a 50 cm wide window. A road runs parallel at a distance of 500 m. What is the cyclist's average speed on this road when the observer sees him at 15 seconds? - MO Z7–I–6 2021
In triangle ABC, point D lies on the AC side and point E on the BC side. The sizes of the angles ABD, BAE, CAE, and CBD are 30°, 60°, 20°, and 30°, respectively. Find the size of the AED angle. - Lighthouse
Marcel (point J) lies in the grass and sees the top of the tent (point T) and, behind it, the top of the lighthouse (P). | TT '| = 1.2m, | PP '| = 36m, | JT '| = 5m. Marcel lies 15 meters away from the sea (M). Calculate the lighthouse distance from the s - Tower's view
From the church tower's view at 65 m, the top of the house can be seen at a depth angle of alpha = 45° and its bottom at a depth angle of beta = 58°. Calculate the house's height and its distance from the church. - Concerning 6294
Two isosceles triangles have the same angle at the apex concerning the base. One has a 17 cm long arm and a 10 cm long base. The second has a base length of 8 cm. Determine the length of his arm.
- Isosceles 6673
Isosceles triangle X'Y'Z' . It is similar to triangle XYZ. The base of triangle XYZ has length |XY|=4cm. The size of the angle at the X vertex is 45 degrees. Draw a triangle X'Y'Z' whose base is 8 cm long. - Calculate 83431
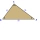In triangle ABC, the size of the exterior angle at vertex C is equal to 126°. The size of the internal angles at vertices A and B are in the ratio 5: 9. Calculate the size of the internal angles α, β, γ of triangle ABC. - Isosceles 7661
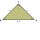The area of the isosceles triangle is 8 cm2, and its arm's length is 4 cm. Calculate the sizes of its interior angles. - Shadow 73354
How long is the shadow of a tree 7.6 m high, and the shadow of a 190 cm high road sign is 3.3 m long? - A boy
A boy of 1.7m in height is standing 30m away from the flagstaff on the same level ground. He observes that the angle of deviation of the top of the flagstaff is 30 degrees. Calculate the height of the flagstaff.
- Mast shadow
The mast has a 13 m long shadow on a slope rising from the mast foot toward the shadow angle at an angle of 15°. Determine the height of the mast if the sun above the horizon is at an angle of 33°. Use the law of sines. - Boat
A force of 300 kg (3000 N) is required to pull a boat up a ramp inclined at 14° with horizontal. How much does the boat weigh? - Inclined plane
On the inclined plane with an inclination angle of 30°, we will put the body (fixed point) with mass 9 kg. Determine the acceleration of the body motion on an inclined plane. - Two-meter 3473
A tree with an unknown height casts a shadow 18 m long at a time, while a two-meter pole casts a shadow of 2.4 m. How tall is the tree? - The shadow
The shadow of a 1 m high pole thrown on a horizontal plane is 0.8 m long. At the same time, the shadow of a tree thrown on a horizontal plane is 6.4 m. Determine the height of the tree.
A meter pole perpendicular to the ground throws a shadow of 40 cm long. The house throws a shadow 6 meters long. What is the height of the house?Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
