Circle + triangle - practice problems - page 15 of 17
Number of problems found: 336
- Magnitude 25411
There is a circle with a radius of 10 cm and its chord, which is 12 cm long. Calculate the magnitude of the central angle that belongs to this chord. - Decagon
Calculate the area and circumference of the regular decagon when its radius of a circle circumscribing is R = 1m - Hexagon A
Calculate the area of a regular hexagon inscribed in a circle with radius r=15 cm. - Nine-gon
Calculate the perimeter of a regular nonagon (9-gon) inscribed in a circle with a radius 18 cm.
- The cone
The cone's lateral surface area is 4 cm², and the area of the base is 2 cm². Find the angle in degrees (deviation) of the cone sine and the cone base plane. (The cone side is the segment joining the vertex cone with any point of the base circle. All sides - Flakes
A circle was inscribed in the square. We draw a semicircle above each side of the square as above the diameter. This resulted in four chips. Which is bigger: the area of the middle square or the area of the four chips? - Circle annulus
There are two concentric circles in the figure. The chord of the larger circle, 10 cm long, is tangent to the smaller circle. What does annulus have? - Rectangular 13731
I have a rectangular trapezoid ZIMA (the right angle at the top of Z. ZIMA = winter in English) ZI-7cm, ZM-5cm, AM-3.5cm, and I have to write the procedure and perform a test in the design task - Circumference 7143
Peter drew a regular hexagon, the vertices of which lay on a circle 16 cm long. Then, for each vertex of this hexagon, he drew a circle centered on that vertex that ran through its two adjacent vertices. The unit was created as in the picture. Find the ci
- Hexagonal pyramid
The pyramid's base is a regular hexagon, which can be circumscribed in a circle with a radius of 1 meter. Calculate the volume of a pyramid 2.5 meters high. - Pavement
Calculate the length of the pavement that runs through a circular square with a diameter of 40 m if the distance of the pavement from the center is 15 m. - Equation of circle 2
Find the equation of a circle that touches the axis of y at a distance of 4 from the origin and cuts off an intercept of length 6 on the axis x. - The circle arc
Calculate the span of the arc, which is part of a circle with diameter d = 11 m and its height is 5 m. - Field with vegetables
The field planted with vegetables has a rectangular isosceles triangle with a leg length of 24 m. At the triangle's vertices are rotating sprinklers with a range of 12 m. How much of the field sprinkler isn't irrigated?
- Ground 8370
The arch has a radius of 3.3 m, a span of 3.25 m, and a height of 20 cm above the ground. What is the length of the arc to reach the ground? - Circle section
An equilateral triangle with side 33 is an inscribed circle section whose center is in one of the triangle's vertices, and the arc touches the opposite side. Calculate: a) the length of the arc b) the ratio between the circumference to the circle sector a - Subtended 83194
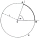A circle k with a center at point S and a radius of 6 cm is given. Calculate the size of the central angle subtended by a chord 10 cm long. - Goat and circles
What is the radius of a circle centered on the other circle, and is the intersection of the two circles equal to half the area of the first circle? This task is the mathematical expression of the role of agriculture. The farmer has circular land on which - Situation 70644
How large is the area colored brown inside a square of side 6 cm if each of the four brown circular segments is from a circle with a radius of the length of the square's side? The length of the circular segments is equal to the length of the side of the s
The base of the regular pyramid is a hexagon, which can be described as a circle with a radius of 2 m. Find the volume of the pyramid to be 2.5 m high.Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
