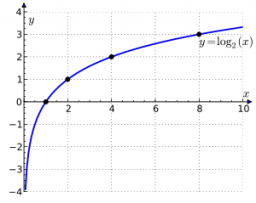
Simplify logarithm expr
Given that logxU + logxV =p and logxU - logxV =q
Prove that U=x^½(p+q)
Prove that U=x^½(p+q)
Correct answer:
You need to know the following knowledge to solve this word math problem:
- algebra
- expression of a variable from the formula
- arithmetic
- exponentiation
- basic functions
- logarithm
- exponential function
Themes, topics:
Grade of the word problem:
Related math problems and questions:
- Linear imaginary equation
Given that 2(z+i)=i(z+i) "this is z star" Find the value of the complex number z. - Prove
Prove that k1 and k2 are the equations of two circles. Find the equation of the line that passes through the centers of these circles. k1: x²+y²+2x+4y+1=0 k2: x²+y²-8x+6y+9=0 - Determine 80662
Given the function y = x² - 4x + 3. Determine all real numbers z such that g(x) = g(-2). - Equation 46771
Insert three numbers between the roots of the equation 4x² - 17x + 4 = 0 so that they form with the given GP numbers
- A circle
A circle relation is given to be x² + y² =16. What is the radius of the circle? - Inequality 7320
Let a, b, and c be positive real numbers whose sum is 3, each of which is at most 2. Prove that the inequality holds: a2 + b2 + c2 + 3abc - Equation 20
In the given equation: 8/9-4/5=2/9+x, find x - Circumscribing 80498
Given is an acute-angled triangle ABC. On the half lines opposite to BA and CA lie successively the points D and E such that |BD| = |AC| and |CE| = |AB|. Prove that the center of the circle circumscribing triangle ADE lies on the circle circumscribing tri - Expression 68414
The expression 3x - [2 - (2x - 1) + x] is given. Determine for which numbers x the expression is equal to 0.
- Intersection 7247
On side AB of triangle ABC, points D and E are given such that |AD| = |DE| = |EB|. Points A and B are the midpoints of segments CF and CG. Line CD intersects line FB at point I, and line CE intersects line AG at point J. Prove that the intersection of lin - X intercept
Given: 3y+2x=-6 Calculate the X-intercept. - General line equations
In all examples, write the GENERAL EQUATION OF a line that is given in some way. A) the line is given parametrically: x = - 4 + 2p, y = 2 - 3p B) the slope form gives the line: y = 3x - 1 C) the line is given by two points: A [3; -3], B [-5; 2] D) the lin - Geometry: 78014
Good day, Even though it is a trivial task, I don’t know how to deal with it. This is analytic geometry: Find all integers a, b, and c such that the line given by the equation ax+by=c passes through the points [4,3] and [−2,1]. Thank you for your answer - Prealgebra ex
Find an unknown number/mixed number N in the given equation: N + 3 1/2 = 6 3/4
- Vectors abs sum diff
The vectors a = (4,2), b = (- 2,1) are given. Calculate: a) |a+b|, b) |a|+|b|, c) |a-b|, d) |a|-|b|. - Coordinates 59763
The vectors v = (2.7; -1.8), w = (-3; 2.5) are given. Find the coordinates of the vectors: a = v + w, b = v-w, c = w-v, d = 2 / 3v - Multiple numerical bases
Find the value of x for which 312four+52x=96ten . Hint: four, x, and ten are the base of the given number.
