See harmonics
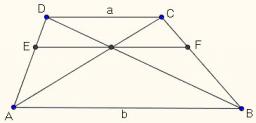
Is it true that the size of the central segment of any trapezoid is the harmonic mean size of its bases? Prove it. The central segment crosses the intersection of the diagonals and is parallel to the bases.
Result
Result
Tips for related online calculators
Looking for calculator of harmonic mean?
Looking for a statistical calculator?
See also our trigonometric triangle calculator.
Looking for a statistical calculator?
See also our trigonometric triangle calculator.
You need to know the following knowledge to solve this word math problem:
Themes, topics:
Grade of the word problem:
We encourage you to watch this tutorial video on this math problem: video1
Related math problems and questions:
- 4-gon
Is it true that a 4-gon whose two sides are parallel and the other two have equal length is a parallelogram? - Trapezium diagonals
It is given trapezium ABCD with bases | AB | = 12 cm, |CD| = 8 cm. Point S is the intersection of the diagonals for which |AS| is 6 cm long. Calculate the length of the full diagonal AC. - Reciprocal
It is true (prove it) that if a > b > 0: (1)/(a)< (1)/(b) - Line
Is it true that the lines that do not intersect are parallel? - Orchard
The route passes the trapezoidal orchard perpendicular to the parallel sides. It is 80 cm wide. The lengths of the bases are in the ratio 5:3. The length of the longer base to the size of the path is in the ratio of 5:6. How many square meters occupy the - Height
Is it true that the height is less or equal to half of the hypotenuse in any right triangle? - Trapezoid 4908
Trapezoid ABCD with bases AB = a, CD = c has height v. The point S is the center of the arm BC. Prove that the area of the ASD triangle is equal to half the area of the ABCD trapezoid. - Trapezoid - intersection of diagonals
In the ABCD trapezoid is AB = 8 cm long, trapezium height 6 cm, and distance of diagonals intersection from AB is 4 cm. Calculate the trapezoid area. - Construction 32971
There is any circle k that does not have a marked center. Use a suitable construction to find the center of the circle k. Try on two different circles. - IS trapezoid
Isosceles trapezoid arm measured 35 cm. Height is 30 cm, and the middle segment is 65 cm. Find the length of its bases. - Calculate 3993
The median of the trapezoid p is 18.6 cm, and the base a = 29.8 cm. Calculate the size of the second base c. - Acute triangle
In the acute triangle KLM, V is the intersection of its heights, and X is the heel of height to the side KL. The axis of the angle XVL is parallel to the side LM, and the angle MKL is 70°. What size are the KLM and KML angles? - Area of iso-trap
Find the area of an isosceles trapezoid if the lengths of its bases are 16 cm and 30 cm, and the diagonals are perpendicular to each other. - Diagonals at right angle
In the trapezoid ABCD, this is given: AB=12cm CD=4cm And diagonals crossed under a right angle. What is the area of this trapezoid ABCD? - Inner angles
The magnitude of the internal angle at the central vertex C of the isosceles triangle ABC is 72°. The line p, parallel to the base of this triangle, divides the triangle into a trapezoid and a smaller triangle. How big are the inner angles of the trapezoi - Powers
Is it true for any number a,b,c,d equality:? (a-b-c-d) 6 = (d+c+b-a) 6 - Medians in triangle
The median of the isosceles triangle has a length of 3 cm. Determine the size of its sides if its perimeter is 16 cm.
