Circle + expression of a variable from the formula - practice problems - page 11 of 13
Number of problems found: 246
- Ratio of squares
A circle is given, and a square is inscribed. The smaller square is inscribed in a circular arc formed by the square's side and the circle's arc. What is the ratio of the areas of the large and small squares? - Pentagon
Calculate the length of a regular pentagon's side, circumference, and area, inscribed in a circle with a radius r = 6 cm. - Horizontal 83148
The bend has a radius of r = 100 m and is inclined at an angle of 20° to the horizontal plane (= tilt angle). What is the safe (the "best") speed to go through this curve? Sketch the picture regarding NIVS, mark the forces, and calculate. - Sphere in cone
A sphere is inscribed in the cone (the intersection of their boundaries consists of a circle and one point). The ratio of the ball's surface and the area of the base is 4:3. A plane passing through the axis of a cone cuts the cone in an isosceles triangle
- From plasticine
Michael modeled from plasticine a 15 cm high pyramid with a rectangular base, with the sides of the base a = 12 cm and b = 8 cm. From this pyramid, Janka modeled a rotating cone with a base diameter of 10 cm. How tall was Janka's cone? - Pentagonal prism
The regular pentagonal prism is 10 cm high. The radius of the circle of the described base is 8 cm. Calculate the volume and surface area of the prism. - Corresponding 59063
Calculate the radius and area of the circular segment if the center angle = 106° and the length of the corresponding circular arc is l = 52 cm. - Moon
We see the Moon from the perspective angle 28'. At the time of the full Moon, the Moon's radius is 1740 km. Calculate the mean distance of the Moon from the Earth. - Disc
The circumference of the disk is 78.5 cm. What is the circumference of the circular arc of 32° on the disc?
- Sidewalk 63134
A 2m wide sidewalk is built around the circular fountain. The radii of the circles that delimit the path on both sides are 4:3. What area in square meters does this sidewalk occupy? - Chord
It is given to a circle k(r=6 cm), and the points A and B such that |AB| = 8 cm lie on k. Calculate the distance of the center of circle S to the midpoint C of segment AB. - Quarter of a circle
Calculate the circumference of a quarter circle if its area is S = 314 cm². - Diameter 5668
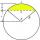The span of the arc is 247 cm, and the height of the arc is 21.5 cm. What is the diameter of the circle? - Circular railway
The railway connects points A, B, and C in a circular arc, whose distances are | AB | = 30 km, AC = 95 km, and BC | = 70 km. How long will the track be from A to C?
- Circular 31441
The circular park has an area of 1600 m². Cross the park, right in its center, leads the trail. What is the length of the trail? - Chord circle
The circle to the (S, r = 8 cm) are different points A, B connected segment /AB/ = 12 cm. AB mark the middle of S'. Calculate |SS'|. Make the sketch. - Steel tube
The steel tube has an inner diameter of 4 cm and an outer diameter of 4.8 cm. The density of the steel is 7800 kg/m³. Calculate its length if it weighs 15 kg. - MO SK/CZ Z9–I–3
John had the ball that rolled into the pool and swam in the water. Its highest point was 2 cm above the surface. The circle's diameter that marked the water level on the ball's surface was 8 cm. Find the diameter of John's ball. - Hexagonal pyramid
Calculate the surface area of a regular hexagonal pyramid with a base inscribed in a circle with a radius of 8 cm and a height of 20 cm.
What is the radius of a circle inscribed in the quarter circle with a radius of 100 cm?Do you have homework that you need help solving? Ask a question, and we will try to solve it. Solving math problems.
